|
The Middle Shogi Manual was compiled by the late George Hodges
This website lists some new insights into these problems
|
From the fact that different manuscripts often give different solutions for the same problem, it was already known that the solutions were in general not given by the composers of the problem. This has apparently created the situation that the more difficult problems were actually too difficult for the authors that incorporated them in their collections, making them produce flawed solutions. The comments given on this in the MSM are not always justified either, though.
All problems were fed to the HaChu engine. This was running in tsume mode with the 'Allow repeats' setting, which puts the obligation to deviate always on the checking side. The 'Promote on entry' setting was switched off, which means that HaChu plays according to the rule that a piece that deferred promotion can promote on later moves that touch the zone, but not on the turn immediately following their deferral (unles they capture). Note that this is different from what the Middle Shogi Manual states, where the condition is that the next move with that piece cannot be a promotion.
Below the cases are discussed where HaChu's analysis disagrees with solutions or comments in the MSM. The issues can consider minor typos, a somewhat faster line, but also complete refutation of a given solution, and spectacular new solutions.
A2 - The MSM claims 2... K-6b is a better defense that would prevent mate, but in fact the mate is much faster in this case: 3. Ln-4c K-7a 4. Lnx5c K-8a 5. Lnx6a K-9b 6. Lnx7b-7a K-10c 7. Ln-9a K-11d 8. Lnx10ax10b mate. While the main line of the solution, including the steps of the 'Lion mate follows', is a mate in 13.
A3 - Only the second solution, apparently given in only one document, is correct. What the other documents give is flawed, as after 4... K-11e there is no mate.
A4 - As the MSM states, the given computer solution is the only correct one.
A5 - The solution given in the MSM is indeed correct, and is a mate in 18.
A6 - The computer solution is indeed the only correct one.
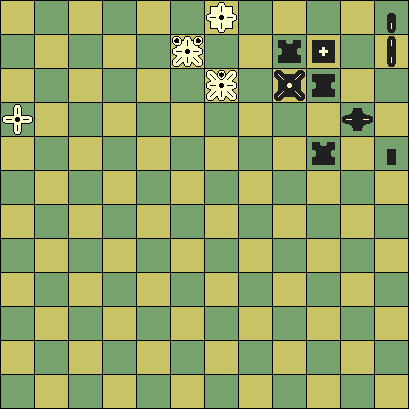
A8 - The second solution, apparently given by only one document, is in any case a correct one. This problem has a bearing on the repetition rule, however. When repeats are strictly forbidden, it is a mate in 14. When the burden to deviate is on the perpetual checker, however, sente cannot use the repetition prohibition to force gote's King to the edge, and the solution gets a much less messy, albeit longer ending for the 'mate that follows' with +G and +DK, which is certainly worth mentioning:
5... K-4a 6. +G-12a K-5b 7. +G-12b K-4c 8. +G-1c K-5d 9. +G-5c K-6d 10. +DK-5e K-7d 11. +G-7c K-8d 12. +DK-7e K-9d 13. +G-9c K-10d 14. +DK-9e K-11d 15. +G-11c K-12d 16. +DK-11e#
It is therefore a pity that this part of the solution is not given, so that we cannot know what repetition rule the authors of the solution were using. The SM on 1d seems especially placed to force the lengthy 'ladder drive' which is necessery when the checker has to deviate, and thus can be considered an indication that the problem composer used to this rule.
The flaw in the first solution is that 3. +DHx4b should have been 3. +DHx!4b (i.e. the Silver should have been taken by igui rather than normally), in which case there wasn't anything to capture on 4b for the Leopard, and the given move 3... K-1c (or K-2c, which loses equally fast) is forced, resulting in mate in 6 (and thus showing how poor defense 2... K-2b really was). So this is probably a copying error in the move notation, rather than a design flaw.
A13 - This problem is flawed: 3... K-k11 refutes the given solution, and this cannot be repaired. The advantage of solving under tsume rules is that you can also with certainty know when a problem is not solvabe: at some point you simply run out of possibilities to check.
However, if we place a Horned Falcon on 3b, rather than a Soaring Eagle, (which have almost identical single-kanji representations, making this a likely copy error), a well-behaved mate-in-6 problem results:
1. Lnx4b Kyx4b 2. Ph-4c= SMx4c (2... +DHx4c 3. +DHx4c G-3b 4. +DHx2b Gx2b 5. +DKx4c K-2b 6. +DKx2c mate) 3. +DKx4d +DHx4d 4. +DHx4d C-3b 5. +DHx3b-3a C-3a 6. FL-5d+ mate
or
1... +DHx4b 2. FL-5d+ +DK-3b 3. +FLx3b Gx3b 4. +DH-2c Gx2c 5. Ph-4c+ K-2b 6. +Phx2c mate
A17 - The MSM remarks that the solution given to this problem is flawed, but does not offer any alternative. Indeed the published solution is totally off, and the first move already spoils the mate (which then is indeed refuted by 2... BT-4e). The correct solution is a very elegant mate in 16:
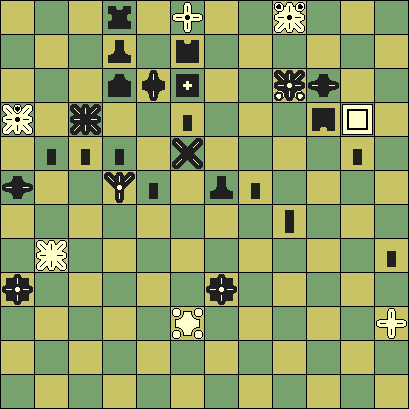
1. +DK-5a K-6d 2. Lnx3c-4b +DKx4b 3. +DKx4b K-6e 4. +DKx4g K-7f 5. Ph-5h K-8e 6. +DK-10g K-9d 7. Ph-7f K-10c 8. +DKx9f-8e K-11b 9. Ph-9d= K-11a 10. +Gx9a Cx9a 11. +DHx9a K-12b 12. Phx7b+ G-9b 13. +Phx9b B-10b 14. +DHx10b FKx10b 15. +Phx10b K-12a 16. +DK-10c mate.
This problem was too difficult for HaChu to solve even after several days of thinking! HaChu seemed, however, to be on the right way, considering the PV it reported for the material-wise most favorable deep checking lines. The problem seems to be that there are many deviations for sente that lead to nowhere, but can continue checking almost forever, when a single slider chases the gote King through an empty region of the board and can do that in a zillion different ways.
Deviations by gote were all rather quickly punished, however. So with some human assistance the computer could prove the mate, by showing that all possible ways for gote to deviate from the tentative PV in the first 6 moves would lead to quick mates that the computer could easily find (mate in 8, at worst). The position that could thus be forced was simple enough for HaChu to determine it was a mate in 10.
A18 - In the sub-variation for 1... K-11a 2. +DK-7a there is the remark that after 2... K-12b there would be no mate, but in fact we have 3. Px12c+ Kx12c 4. +DKx12a DKx12h 5. FKx12h mate.
A19 - The Forsyth notation for this problem in the MSM had a FL in stead of a +FL on 4b.
A20 - This problem can be solved with the Pawn on 3e as well as 3f, in the latter case even against the supposedly better defense 1... Ky-7a:
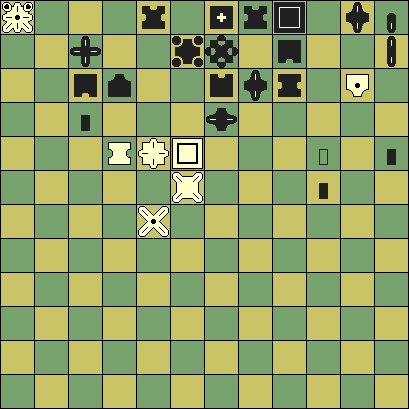
1. +DKx8a Ky-7a 2. DK-8c+ K-6b (2... Gx8c 3. Lnx8c K-5b 4. Lnx7bx7a mate) 3. FL-8d+ SMx8d 4. +DKx8d K-5b 5. +DK-7d K-6b 6. +DK8a-8d K-6a 7. DHx4c+ Lnx4c 8. +Bx4c BT-5b 9. L-8c Gx8c 10 +DK7d-8c mate.
The position of the Pawn matters in the sub-optimal defense
2... K-5b 3. DHx4c+ Lnx4c 4. Bx4c K-4a 5. Lnx6dx5d VMx5d 6. +DHx5d K-3a 7. +DHx3f {a Pawn on 3e would have been blocking this check} K-4a 8. +P-3b BTx3b 9. +DKx3b mate
It turns out this cannot be repaired after the first move, so that what is sub-optimal defense with the Pawn on 3f becomes an iron-clad refutation of 1. +DKx8a when it is on 3e. The latter thus needs a completely different line:
1. +DHx4c+ S-5b 2. DK-8c+ K-5a 3. +DHx4bx4a Sx4a 4. +DK8cx8a Ky-7a 5. +DKx7a Phx7a 6. +DKx7a K-4b 7. +DKx5c BTx5c 8. G-3c Kx3c 9. Lnx6dx5c K-2d 10. Ln-4d K-1c 11. Ln-3d mate.
This line also works with the Pawn on 3f, but is sub-optimal there, as it is mate in 11 rather than 10.
A21 - The given solution can be improved to a mate in 10 by: 7. +DH12a BT-10a 8. +DH-12d K-9b 9. DK-8c+ mate.
A22 - The first move of the given solution already spoils the mate. The correct solution is a simple mate in 5, though: 1. +DK-5d K-6a 2. Lnx9d-8c Cx8c 3. +DK-7b BTx7b 4. +DH-9a BT-8a 5. +DHx8a mate.
A23 - The given solution can be improved to a mate in 10 by: 7. +SM-6a BT-5a 8. FK-4c +DK-4b 9. Phx4b+ mate, which makes 5... K-4a better defense, as in the line given for that the mate can be further delayed by 9... BT-7a 10. +SMx7a mate.
The problems pointed out as defective in the MSM, (A17, A20 and A22), could all be solved. It was thus not so much the problems that were defective, but the solutions provided with them in the historic documents. On the other hand, one problem assumed to be correct turned out to be flawed (A13), in the sense that there doesn't exist a solution. A substitution error (Eagle for Falcon) during copying was pointed out as a possible cause.